Necessary Financial Math

Math. Love it or hate it, if you want to understand your finances you’ll need to learn a little math. For example, in a previous post I told you that if you saved $503 a month, you could have 1 million dollars at retirement. What if you didn’t believe me – or more importantly, what if you could only save $300 a month? Perhaps you think my 6% return on investment was too generous and want

to see what would happen if you earned only 5%? With a few simple tools you could easily do these calculations and more. Fortunately, you really need to understand one simple concept for virtually every calculation you’ll need: The Time Value of Money (TVM).

The time value of money

How simple is the TVM? Ok, what if you lent a close friend $100 today and they offered to give it back to you tomorrow or in one year – which would you chose (assuming they will not abscond)? Most of us would take the money tomorrow. Why? Well, a dollar received in the future is worth less than a dollar received today. There are “opportunity costs” associated with lending someone else your money. There is inflation – just look at how much less education your money buys each year. Perhaps most importantly, there are investment costs. If you had the $100 you could invest it and made more money.

What if your friend offered to give you $100 tomorrow or $105 dollars in one year – would you take it? Friendship aside, how could you decide if this was a good deal? The answer is the TVM calculation. The actual formula looks like this:

TVM Formula

FV = PV x [ 1 + (i / n) ] ^{(n x t)}

Where:

• PV = Present value of money
• FV = Future value of money
• i = Annual interest rate
• n = number of compounding periods per year (usualy 12)
• t = number of years

Calculations made simple

Fortunately you really don’t have to remember the formula or be able to calculate it by hand. When I need to work a TVM calculation I reach for my handy HP 19BII financial calculator. I would recommend that anyone serious about their money should pick up a calculator capable of standard TVM calculations. A good quality used HP17B can be found on eBay for ~$20 or HP10B for about $10. Either will provide you with all the horsepower you will probably ever need. Personally, I prefer the HP17B.

But wait- it’s the 2000s. There should be an app for this! In fact there is. You can download an app for free that can make many (but not all) the calculations you’ll need right Here. If you download it you can follow along while we answer the question: Is your friend’s offer of $105 in 12 months a good deal? Well let’s look at the app mentioned above. If we go to “TVM Calculator” and enter -$100 for present value, 6% for interest and 12 periods (months) the problem is set up like this:

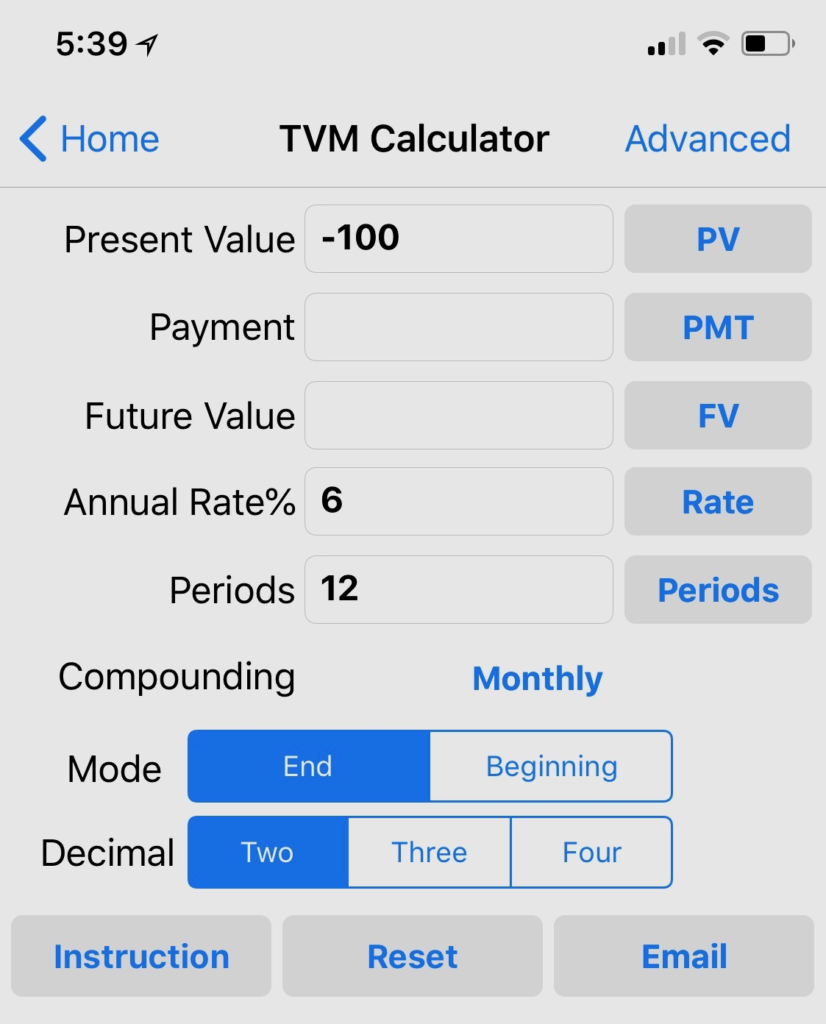
We enter the $100 as a negative because we are giving money up today. We used 6% because we expect that we can earn 6% by investing the money. 12 represents the number of compounding periods per year. By pressing the “Future Value” button we see that if we invested the $100 today and were able to earn 6% over the course of the next year, we would expect to get ~$106 back in the future (note the $106 is a positive number because we are receiving it as income). Please see below:

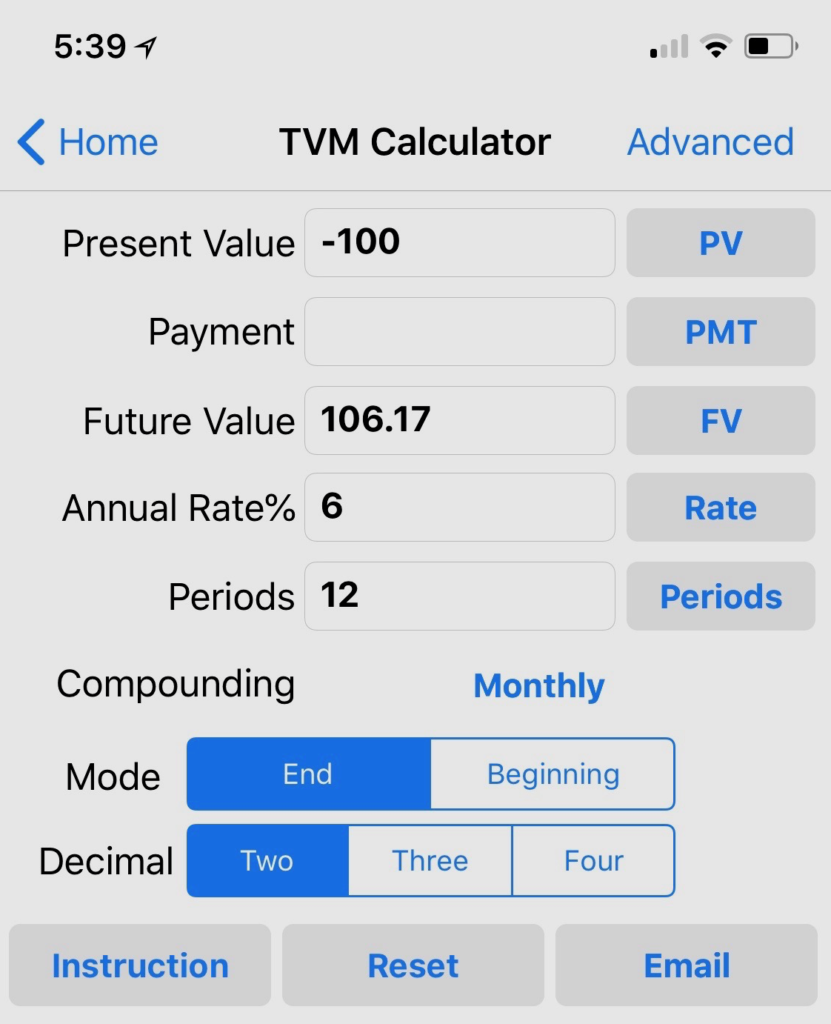
Since we could get $106 by investing our $100 and our friend is offering us only $105, our friend’s offer isn’t very good! But how does this help us calculate how much we will have at retirement if we save every month? For this we need to explore the concept of an annuity.

What about an annuity ?

An annuity is simply a series of equal cash flows, equally distributed over time. Your mortgage is an example of an annuity. If you save every month for retirement, that’s an annuity as well.  The formula is unnecessarily complicated, but its just another TVM calculation and we have technology! Remember the previous example of saving $503 every month from your 25^th birthday to your 65^th? You could set it up as below:

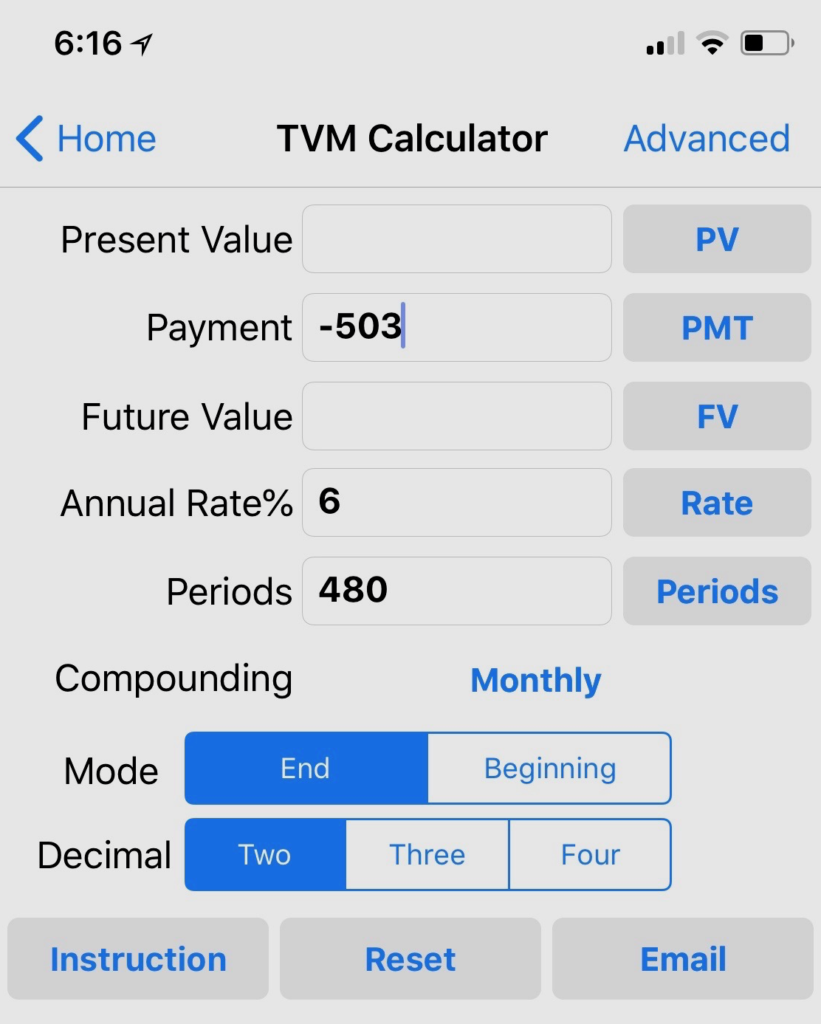
The payment is negative because we are paying it out each month. There are 480 months between now and retirement (40 years X 12 months in a year) so 480 is in “periods” and 6% is what we think we can earn on investments. Pressing the FV button we see that we would expect to have ~$1 million dollars at retirement. (notice the $1 million is positive because it comes back to us as income). See below:

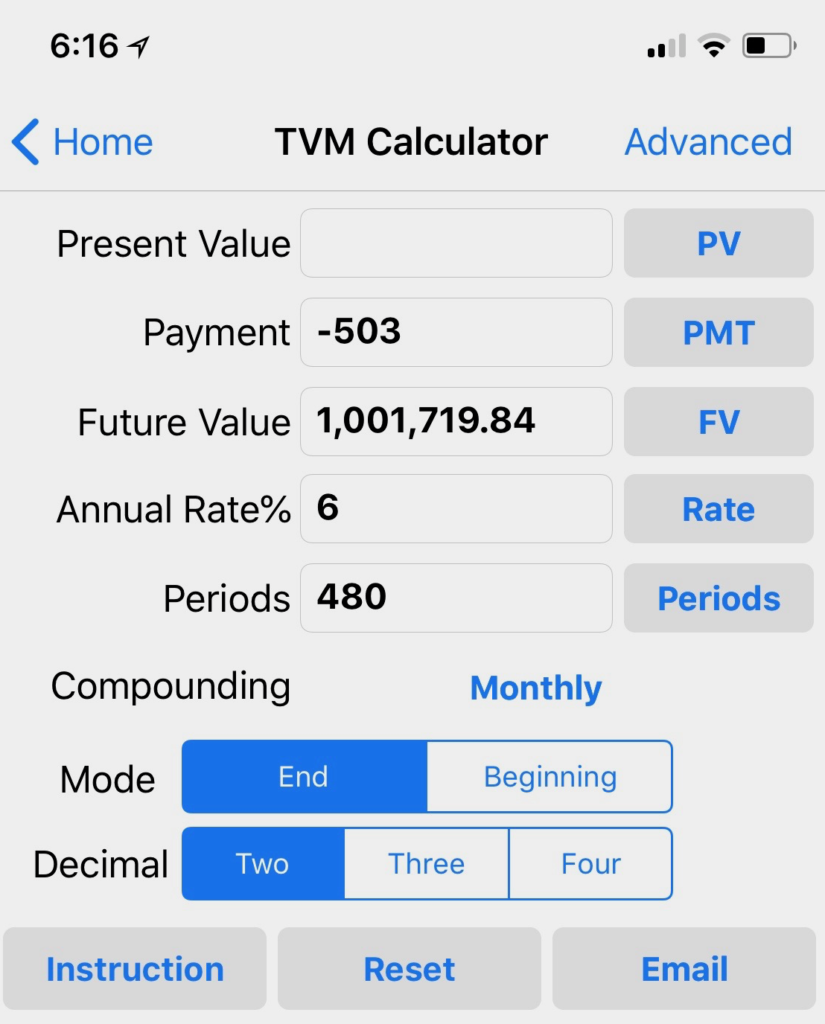
But what if we can only save $300 a month? Simple! We set the problem up as below:

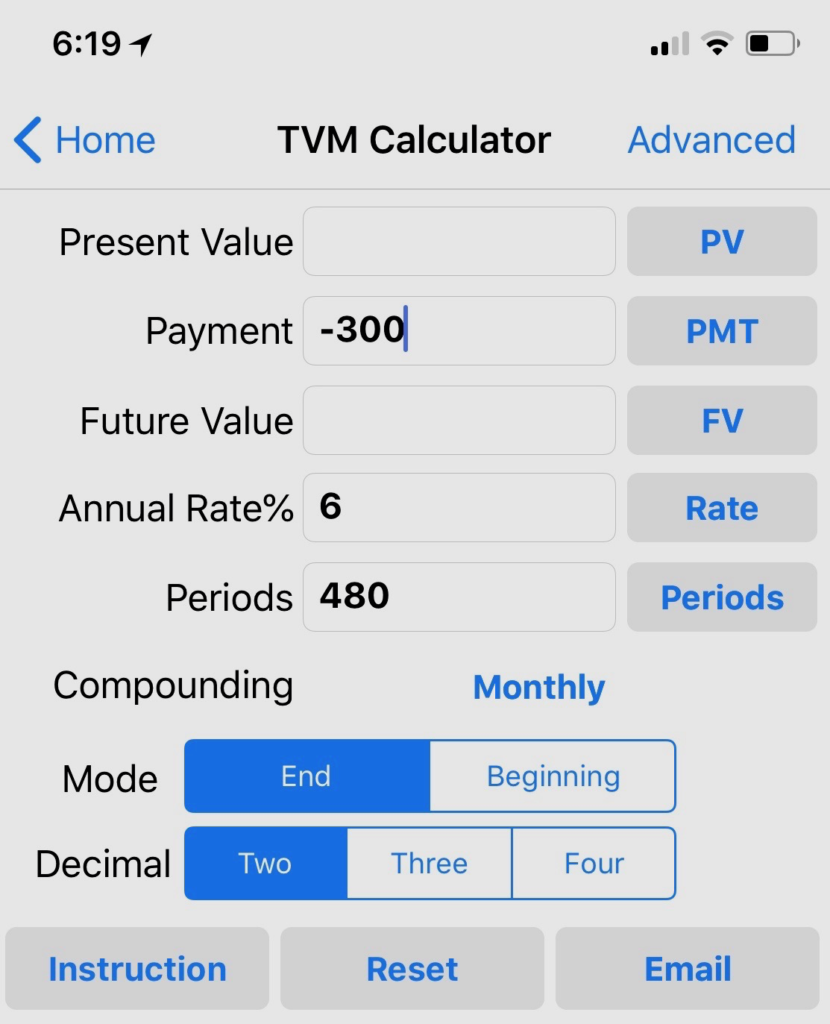
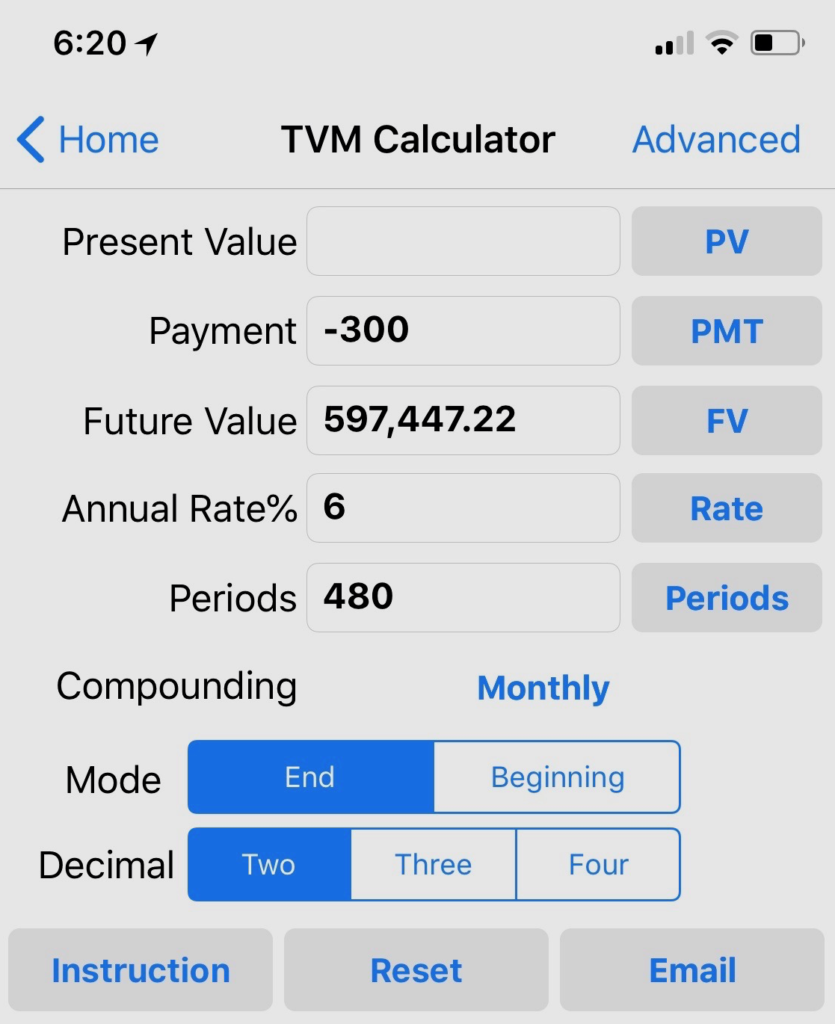
By pressing FV we see that we will accumulate almost $600k in wealth by the time we retire!

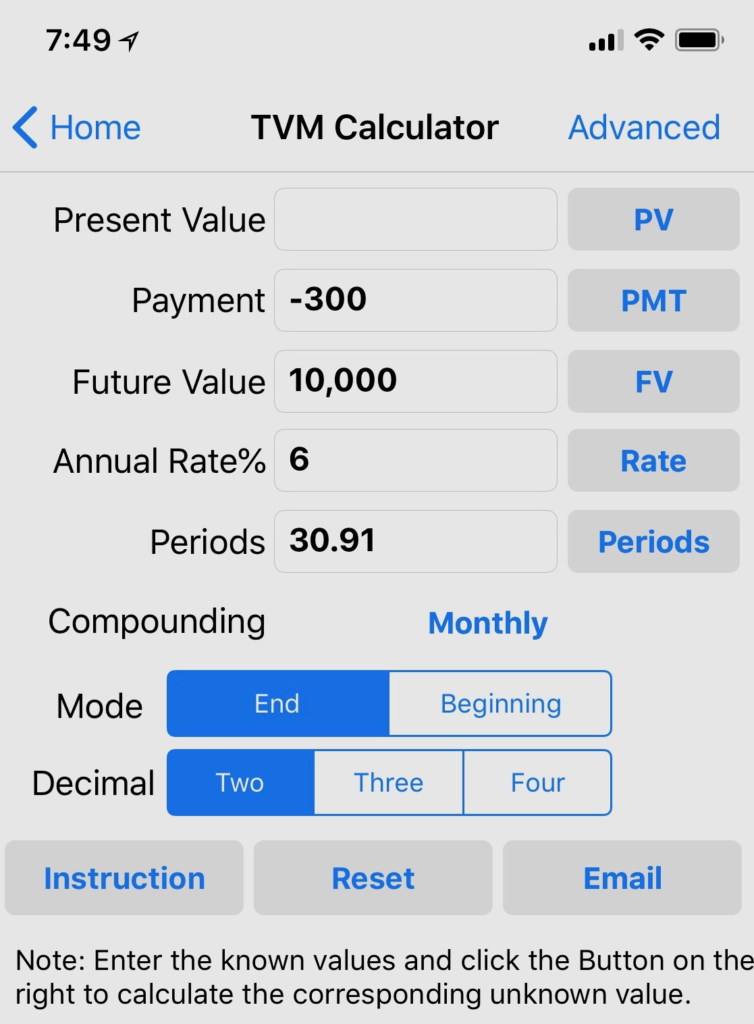
What if we knew we could save $300 a month and expect to earn 6% – how long would it take you to accumulate $10,000? We can set up the problem by entering -300 in payment, 6% as the annual interest rate and $10,000 in future value.

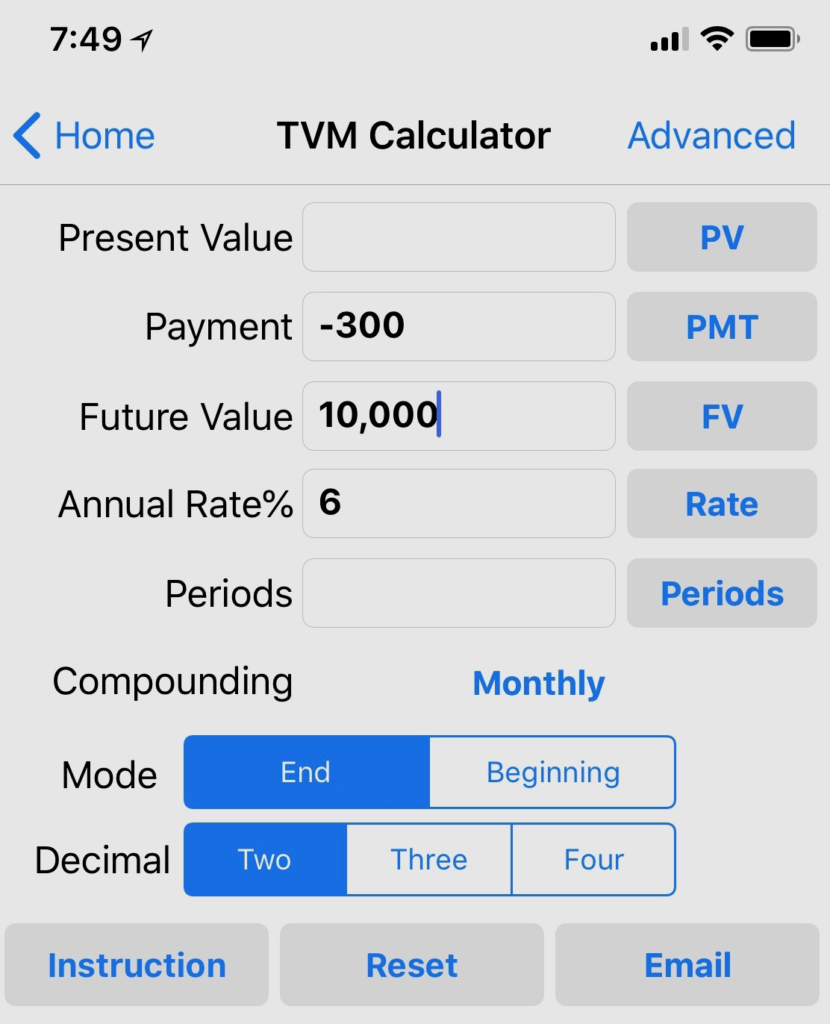
By pressing “periods” we get ~31 periods or months.

One final thought……

So that’s it! You know virtually all the math you need to plan your financial future! Simple – it really is! In the future we will explore further examples ! 

Remember, I would appreciate any feedback especially on how to improve these examples, so please remember to drop me a note!